OmniSim

Modelling Gratings with RCWA

Simulation of infinitely periodic gratings with OmniSim RCWA software

We will show you here how to use OmniSim's RCWA engine to model an infinitely periodic diffraction grating illuminated with oblique incidence.

Different approaches can be used to model diffraction gratings:

• You can use the RCWA engine or with the FETD engine with Bloch boundaries to model the infinitely periodic case.

• You can use the FDTD and FETD engines with absorbing boundaries to model a grating of finite length with an incident beam of finite width.

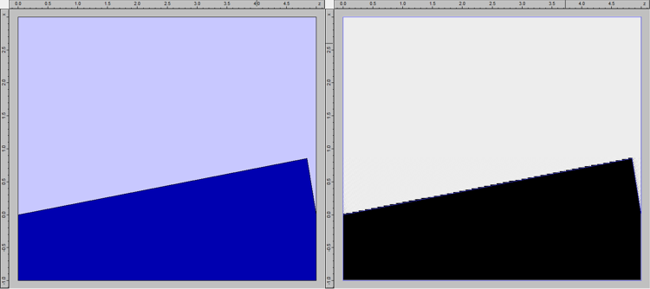
The RCWA approach is ideal as it allows you to calculate how much light is coupled to each diffraction order as well as how much light is absorbed; you would not be able to obtain this information with precision with an FDTD or FETD engine.

Designing the unit cell for the infinitely periodic grating

When modelling the infinitely periodic grating, we only need to consider a single period of the grating. The design is shown below on the left-hand side. The dark blue region corresponds to the silver grating, and the pale blue region to air. On the right-hand side you can see the structure as it is rendered by the Fourier series of the RCWA engine.

  

Unit-cell of the infinitely periodic diffraction grating designed in OmniSim
(left) designed in the graphical user interface (right) discretised by staircasing and Fourier Series

The design settings are given in the table below.

  

Schematic view of the grating; k is the wave-vector of the illumination,
the diffraction orders for the reflected beam are shown for theta = 20 degrees

Modelling the diffraction grating with RCWA

The structure is illuminated with a tilted plane wave excitation; the RCWA engine allows you to control the orientation of the illumination by rotating the plane wave both in-plane and out-of-plane.

You can see below the Ex component of the nearfield profile resulting from the RCWA simulation.

  

Amplitude of the Ex component of the near field shown over three unit cells

  

Real parts of the Ex and Ez components of the near field;
note that these fields are pseudo-periodic i.e. periodic with a phase shift

RCWA is a modal method and it allows us to calculate how much power is coupled to each diffraction order with precision. The results obtained after the RCWA calculation are given in the table below.

Most of power is coupled to the diffraction of order m=1, and a small amount is coupled to the other orders of diffraction. The remaining 5.01% of power was absorbed in the metal. 

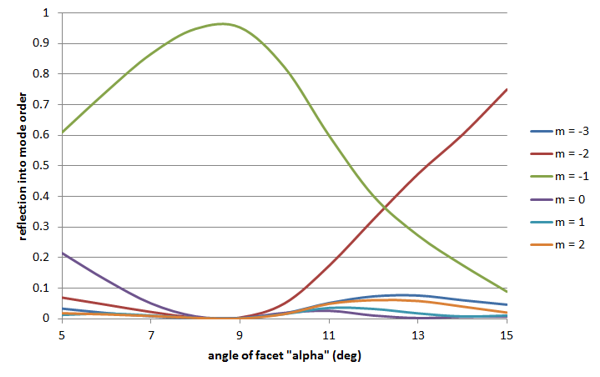
The convergence of the results was studied when varying the number of modes used in the RCWA calculation and the number of layers used to discretise the tilted profile of the grating. The RCWA Engine was able to provide initial results in under 10s and converged results with a high accuracy in 2.9 minutes on a computer with a dual-Xeon E5-2630v3 CPU.

Scanning the orientation of the facets

We used the RCWA scanner to study the variation of the reflected power in each diffraction order when varying the orientation "alpha" of the facet, the orientation "theta" of the illumination being kept constant. The results are shown below. The order m = -1 (closest to the direction of the incident beam) receives maximum power for an angle of 8.7 degrees; as the tilt of the facets increases, an increasing amount of light couples into m = -2 (closer to the grating plane).

  
Amount of power reflected in each diffraction order as a function of the angle "alpha" defining the orientation of the facet

Modelling Echelle gratings and WDM devices

If you are interested in modelling diffraction gratings for use in echelle gratings and WDM Devices, please have a look at Epipprop, our unique echelle grating model.